Back in 2018 I wrote a 3 part blog series for NCTM on Units Coordination (found here: Part 1, Part 2, Part 3 and Part 4 on my blog). At that time it was still relatively new learning for me. The blog series allowed me to pull together everything I had been working on into a (hopefully) coherent form. Here it is 6 years!!! later and I am still learning, reading research, and trying to leverage my understanding to help students grow in their ability to coordinate units. The one thing I am confident about is that students' ability to coordinate units impacts their ability to do math at all levels.
Recently there have been a few things that have crossed my path that prompted this blog post.
This post by Michael Pershan resonated with me. I grabbed a book off my shelf today to look for inspiration for a project and there was a post-it on one of the pages that said, "Why does the claim seem to stick and not the reasoning?" So many students are taught conceptually. They notice patterns. They generalize. These are all the things we want students to do, but as they move forward through the years all they remember are rules. They generalize a pattern seen in written notation, but they don't deeply understand the number relationships that exist in that pattern. So how can they extend that into the world of variables?
A quote that really hit me hard recently comes from this article from Anderson Norton.
Steffe (2007) has estimated that, by the end of fifth grade, 30-50% of students operate at Stage 1 (e.g., Cody), where the remainder of the population is comprised of students operating at Stages 2 or 3. Coordinating additional units, i.e., moving from one stage to the next, requires a substantial reorganization in students’ ways of operating, which can take as long as two years (Steffe & Cobb, 1988; Steffe & Olive, 2010)
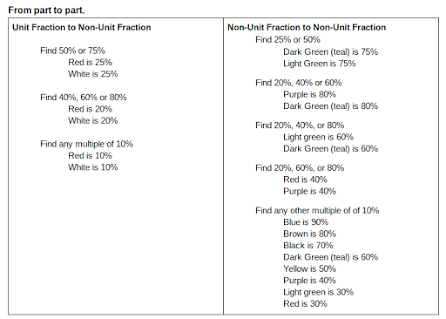
If you haven't read the other blog posts, students at Stage 1 do not have multiplicative reasoning. In a problem like 8 x 4 a student needs to recognize that there are 8 groups of 4. 4 being four individual items and a unit of 4. Multiplication strategies will chunk the 8 into small groups, such as 5(4) + 3(4). A student then has to unitize 5 groups of 4 as being a unit, groups of 4, and individual items all at the same time. A student at a Stage 1 does not have the ability to coordinate all of those units. A student at Stage 2 might not be able to either.
Think about what this means for algebra. The same article references research that found students operating at a Stage 1 "do not readily represent an unknown length with a letter". How could they? They only understand the number 4 as 4 individual items and need to build groups of 4 with individual items in order to see there is a group there. How can an x represent anything other than 1?
Students at a stage 2 can use letters to represent unknown lengths, but it is the coefficient they struggle with. They understand 4 as four individual units and as a unit of 4. They do not see multiple groups of 4 as a unit, and that is the multiplier in an expression like 4x.
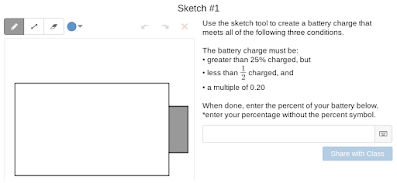
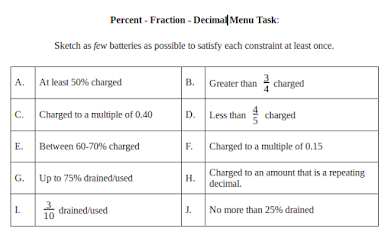
So what is our path forward for these students? The researchers will tell you that to improve a student's ability to coordinate units you need to give them a task that has more units than they can handle and have them solve it "with activity" (manipulatives, drawings, etc). We need to build this idea of units in a concrete way. When I think about the different representations of algebra I have some initial thoughts:
Hanger Model: does a great job helping students understand equality and the balance that exists in an equation. For stage 1 students to understand a shape on the model with a value higher than 1, you might need to pull out some connecting cubes or counters to build what is in the model for them to see all the individual units and start to unitize the group.
Algebra Tiles: will help students at a Stage 2 understand the coefficient. A connection students seem to miss is that 4x is multiplication but also repeated addition (x + x +x + x). It is important to facilitate conversations about this because the written notation shows multiplication but algebra tiles show addition.
Algebra Pan Balance or Cups (see Part 3 above): these are probably the best representation for students at stage 1 because the variable is unknown but there are individual items in the container. This will help students start to see that the variable as a unit (cup) but also individual items.
I have also used Splat by Steve Wyborney for solving equations. Similar to using cups but it is a digit representation. I shared this work at the Wisconsin Math Council virtual conference in 2021.
So to circle back to Micheal Pershan's post, I believe it is the number relationships at the heart of the units coordination work that we need to pay attention to, support, and build for students. We need to keep circling back to the representations and check in on the student's ability to describe, explain, and create those representations. We need to carefully select representations that help students unitize and engender more sophisticated understanding. Our goal is to help students understand math in a way that they can transfer it and apply it to new problems. The more levels of units they can work with at one time will impact how they interpret and understand new problems because not all 3s are created equal.