Percents with Cuisenaire Rods

I was prepared for today.  I had an intervention with a 7th grader.  We have been building his units coordination and multiplicative reasoning through fraction work.  I had it all planned out based on some success we had seen the session before.  Then he came in worried and wanted to work on an assignment from class.  Knowing that I could work some of our fraction conversations into the assignment I pushed my plans aside and we looked at his homework.  

The assignment was on finding percents using a double number line to help think about the number relationships in the problem.  A double number line is an especially nice representation for this student because it allows him to coordinate and keep track of the units (percent and value, parts and wholes) in a visible way.  

I don't remember the exact problem, but it was about basketball free throws.  12 were made and that was 75% accuracy.  It was asking how many shots were attempted.  

Here is our work as we were discussing.  

The assignment gave an open number line.  He was able to correctly place 75%.  Then wanted to add 50% as 6, because 6 is half of 12.  

As we worked on this problem together and I thought about what he knows and can do with fractions, his confusion did not surprise me.  In fact, I had a huge revelation about percent problems like this, their connection to fraction understanding, and the role of units coordination.  

I am going to frame this in terms of fractions and then you can re-read it replacing the percents into it and think about it through that lens.  

So the student is given the fact 3/4 is equal to 12.  The student needs the understand that 3/4 is made up of 3 - 1/4 pieces (which to a middle schooler is a lot clearer than knowing 75% is made up of 3 - 25% pieces).  

So the 3/4 piece needs to be considered 1 unit that needs to be partitioned into 3 pieces.  So the 12 is divided by 3 to get 4.  

Then the 1/4 piece needs to be considered 1 unit that is iterated 4 times to make up the whole.  

Throughout all of this one whole is a unit that has existed to help us know the size of each piece (our denominator or percent).  

If you kept track through that, this problem has 3 levels of units.  The whole, the unit fraction, and the non-unit fraction.  That doesn't even include the units on the top of the number line, which also has 3 levels of units being worked with in tandem with the percents.

So to do this problem the student needs to be able to:

*Understand fractions and/or percents as measurements.

*Be able to partition and iterate fractions and/or percents.

*Coordinate 3 levels of units.  

Now that I was seeing a clearer connection between fraction development and percents my brain immediately went to Cuisenaire rods.  I have used rods a lot in fraction work and they are a great concrete way help students with all 3 of those bullet points above.  

Mark Chubb (inspired by Dan Meyer) has talked about how important it is to move students up and down the ladder between concrete, representational, and abstract.  Cuisenaire rods seem like a great way to take a few steps down the ladder to make this problem more concrete.  

Because I was also thinking about the levels of units I started to think about the different layers in this problem.  For example: If the problem had given 25% (or 1/4), then there would only be 2 units to coordinate, the unit fraction and the whole.  

It also made me wonder if it is easier for a student to be given 25% and find 100% or be given 100% and find 25%.  I suppose that depends on where the student is in their understanding of partitioning and iterating.  

I decided to pull out my Cuisenaire rods and start thinking about it.  

Here is the document I came up with.

First I stripped away the top part of our double number line.  I wanted to focus on the percent and not the value attached to it.  This is much like the fraction work I have done with the rods.  Only this time students will be partitioning and iterating percents, and hopefully getting a better understanding of percents as measurement.  Many students tend to have a better part-whole understanding of fractions, so it makes sense that they have a better part-whole understanding of percents.  I am not sure I ever really thought about percents having both a part-whole and a measurement model.  (Seems so obvious, right?  Usually the big aha's are. How did I not see this before?)

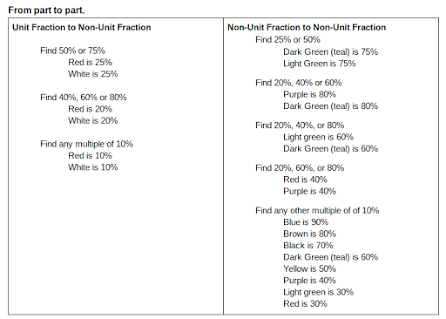
The first section starts with 100% and has the student find a part.  I separated it into finding unit fractions (are unit percents a thing?) and non-unit fractions because it is the difference between coordinating 2 levels of units and 3.  The left column should be easier for students to think through as they develop their understanding.  

Then I came up with options for starting with the part and determining the whole or 100%.  Again, breaking it apart into unit fractions and non-unit fractions.  If given 25%, students would iterate that piece 4 times to find 100%.  If students are given 75%, they have to determine 25% and either compose the 2 parts or iterate the 25% to get to 100%.  

Then I noticed in my fraction work that I have some where students are given a fraction and have to find another fraction.  So I wanted to create the same with percents.  Students are never specifically asked about the whole (100%), but they still need to be aware of it as they think about the number relationships.  I think that means that all of these require coordinating 3 levels of units, but maybe because it's percents it doesn't.  (UC friends, let me know if I'm wrong about that.)

Given 20% find 60%.  This requires iterating the 20% 3 times to find 60%.

Given 75% find 50%.  This requires a student to know that 75% can be partitioned into 25% pieces, 2 of which make 50%.

All of this percent work could definitely be done as part of understanding percents and fraction, decimal, percent conversions.  In the future I will do this type of activity earlier in the school year, before students have to find percents of a number.  

Then to extend this work to what my student was working on I can simply attach a value to the rod a well as a percent.  Here are some examples in each category:

I spent a lot of time by myself playing with the Cuisenaire rods as I processed all this information.  I learned so much and thought about percents in a way I hadn't before. The document I came up with is simply a cheat sheet for me.  I have found that I cannot think these things through on the fly.  If I try I end up choosing a rod as a percent that doesn't work with the other rods that are there - although I should note that the "piece" that students find does not have to be just one rod.  It opens up the possibilities if students can combine rods together to make the needed lengths.   Jo Boaler actually has a nice activity like that in her Mindset Mathematics Grade 6 curriculum.  It uses fractions so would have to be converted to a percent activity for my purposes here. 

Of course it doesn't stop there.  Mark Chubb, as a true math coach, got me thinking:

Kids totally need to do what I did today.  There was so much great relational thinking.  

• What rods can I choose?  
• Which rod won't work as 50%? 
• Which rod doesn't have a 50% rod to go with it?  
• What other percents can I make with 20%?

Obviously these are all questions I can ask students as we explore together.  

When I have done fraction activities, I have had students make up their own puzzles for us to solve with the rods. Then we share them.  This would also be a great way to engage students in the exact task I was doing today.  Since my document is just a cheat sheet I won't be using all the options with the students.  That leaves options for them to find through exploration.  

If you made it to the end of this very long post, I would love to know your thoughts.  

• What might percent increase problems look like?
• What other types of percent problems am I missing? 
• What struggles do you see getting in the way of students being successful with percents?